The Most Influential
People in History

Pierre de Fermat / fehr-MAH /
1601–1665
French Lawyer and Mathematician
Mathematics Ranking 25th of 46
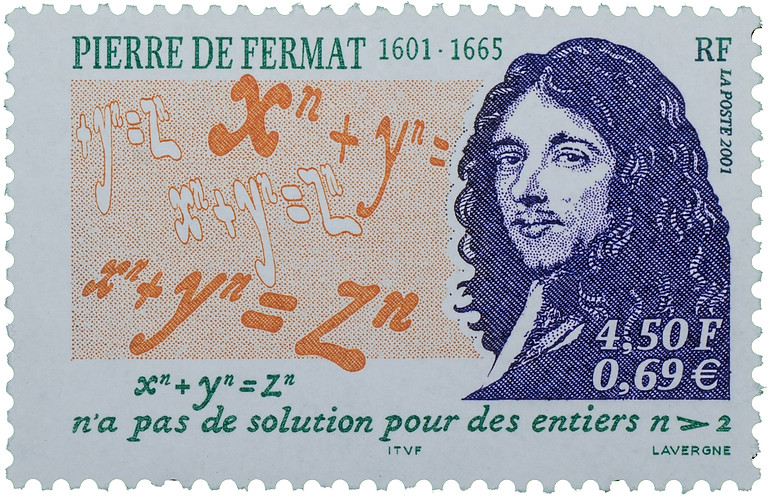
“Fermat’s Last Theorem” on a French stamp.
Fermat made significant contributions in several areas of mathematics with his seminal work in number theory being paramount.
Mathematics–Pre-Calculus, Number Theory
Fermat made many discoveries about integers, for which he is seen as the founder of the theory of numbers. Euclid’s Elements contains some number theory, but Fermat’s work was more comprehensive, so he is credited as the founder.(1) Though Fermat is considered the founder of number theory, he had studied from a great foundation called the Arithmetica by the Greek Diophantus of Alexandria (c. 250). In 1621, Claude Gaspar Bachet de Meziriac translated the Arithmetica into Latin and finally made available after almost 2,000 years a comprehensive text on number theory, equivalent to Euclid’s Elements for geometry.(2) Fermat’s study of the problems of finding tangents to curves, finding areas under curves, and maxima and minima led directly to the general methods of calculus introduced by Isaac Newton and Gottfried Leibniz.
Mathematics–Theory of Probability
The modern theory of probability is usually considered to begin with the correspondence of Blaise Pascal and Pierre de Fermat in 1654, partially in response to the gambling questions Antoine Gombaud, the chevalier de Mere, presented to Pascal.(3) Gombaud could not understand why he was losing when he bet even money that double sixes would turn up at least once on average in every twenty-four rolls of two dice. The answer is once in 24.6 rolls, which means, since dice cannot be rolled a fractional number of times, that someone could only win this bet at even money if the dice were rolled at least 25 times. Later, insurance companies adopted Pascal and Fermat’s work for actuarial tables of sickness and mortality.
Mathematics–Number Theory (“Fermat’s Last Theorem”)
In Fermat’s copy of Diophantus’s Arithmetica, in the margin next to Problem 8, we discover what is called “Fermat’s Last Theorem.” The theorem is written out in Latin.
Cubem autem in duos cubos, aut quadratoquadratum in duos quadratoquadratos, et generaliter nullam in infinitum ultra quadratum potestatem in duos eiusdem nominis fas est dividere.
It states the proposition that if n is greater than 2 then there is no integer whose nth power can be expressed as the sum of two smaller nth powers. According to Fermat, there appeared to be no three numbers that would perfectly fit the equation xn + yn = zn, where n represents any number greater than 2.
(Note that Pythagoras’s equation for right triangles is x2 + y2 = z2 and has an infinite number of solutions.)
1

This Czechoslovakian stamp from 2000 illustrates Andrew Wiles’s confirmation of “Fermat’s Last Theorem” in 1995.
The conjecture (of which Fermat noted that he had “a truly wonderful proof but it could not be fit into the margin”) has been demonstrated by calculation to be true for very many possible values of n. Fermat did prove for n=4 which was in a manuscript discovered later, after his death—but the other cases, he almost certainly did not know how to prove. Euler also proved Fermat’s last theorem for n=4 in 1738 but it took another 30 years for him to prove that there were three integers that satisfy this equation for the exponent 3. His letters to Goldbach show that the proof cost him a great deal of effort because it called for the introduction of new methods.(4) For the exponent 5 there is no solution. Three mathematicians, Sophie Germain, Gustav Lejeune Dirichlet, and Adrien-Marie Legendre each contributed to the solution. In 1832 Dirichlet went back to the problem and showed that you cannot get a solution when the exponent is 14. There is no reason to check the case where the exponent is 6 because if we cannot have a solution with the exponent 3, then we also cannot have a solution with the exponent 6. The exponent 7 was solved by Gabriel Lamé in 1839.
At this point, significant progress was made by at least eleven people(5) until, in 1993, a general proof was announced by the Princeton-based British mathematician Andrew Wiles.(6) However, it took another year to fix a major flaw in his proof, which was eventually published as two papers totaling 130 pages in the Annals of Mathematics (May 1995). Wiles had first seen Fermat’s theorem in 1963 when he was 10. Later, after becoming a mathematician, he decided in 1986, at age 33, to devote much of his free time to solving Fermat’s devilish problem. He still had to fulfill his duties as a professor of mathematics at Princeton by teaching classes, but avoided conferences and lectures, secretly working in the attic of his house. By 1994, after an eight-year ordeal, he had synthesized almost all the breakthroughs in twentieth-century number theory and incorporated them in one giant proof. He had created completely new mathematical techniques and combined them with traditional ones in ways that had never been considered possible. In doing so, he had opened up new lines of attack on a whole host of other problems. According to John Coates, his graduate adviser, Wiles’s proof of Fermat’s claim “revolutionized number theory in one fell swoop.”(7) As a last note, Wiles collected $50,000 for proving Fermat’s theorem.
Footnotes:
(1) Judy Pearsall and Bill Trumble (editors), The Oxford Encyclopedic English Dictionary (New York, 1996), p. 513.
(2) Simon Singh, Fermat's Enigma (New York, 1997), p. 50.
(3) Carl B. Boyer, A History of Mathematics, 2nd edition (New York, 1991), p. 363.
(4) Isabella Bashmakova and Galina Smirnova, translated from the Russian by Abe Shenitzer, The Beginnings & Evolution of Algebra (Washington, D.C., 2000), p. 129.
(5) David Bressoud, The Queen of the Sciences: A History of Mathematics – Lecture Transcripts, Lecture 22, (Chantilly, 2008), pp. 163-170.
(6) The Oxford Encyclopedic English Dictionary, p. 513.
(7) Singh, p. 279.