The Most Influential
People in History

Apollonius of Perga / ahp-uh-LOH-nee-uhs /
c. 260–190 B.C.E.
Greek Mathematician
Mathematics Ranking 12th of 46

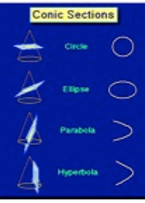
Apollonius of Perga was the first to use the terms ellipse, parabola, and hyperbola for three different classes of curves in his surviving work the Conics (194 pages), a classic treatise on conic sections, superseding all earlier works on the subject. Conics is one of the greatest scientific works from the ancient world.(1) Of the eight books into which the Conics was divided, four survive in the original Greek, three others in Arabic translation only, and one is lost. Apollonius defines his curves as intersections of a plane with a double cone. He distinguishes between the three possibilities respectively producing the parabola, the ellipse, and the hyperbola, so named from characteristic properties of the curves expressed in terms of Pythagorean 'geometrical algebra'. The later books deal with the properties of tangents, normals, asymptotes, conjugate diameters, and evolutes of conics. Harmonic properties and rectangle theorems are also covered.
The ellipse is the figure that represents the orbits of the planets. Much later, in the early 1600s, Johannes Kepler discovered this fact. The parabola approximately represents the path of a cannonball that was not realized until the early 1600s by Galileo Galilei. The hyperbola is used to describe the expansion of gas in an internal-combustion engine.
Apollonius, who studied at Alexandria, was involved in the contemporary development of planetary theories, though here his exact contribution cannot be defined with certainty. It was probably he who generalized the hypothesis of epicycles (devised to represent the apparent motions of Mercury and Venus) to account also, by means of eccentric orbits, for the observed movements of the outer planets, Mars, Jupiter, and Saturn.
Footnotes:
(1) Encyclopaedia Britannica, Micropaedia, Volume 1, 1993, 15th Edition, p. 485.
Key Reference: T.L. Heath, A History of Greek Mathematics, volume 2, 1921.
1